How to work out the shaded area of a rectangular shape
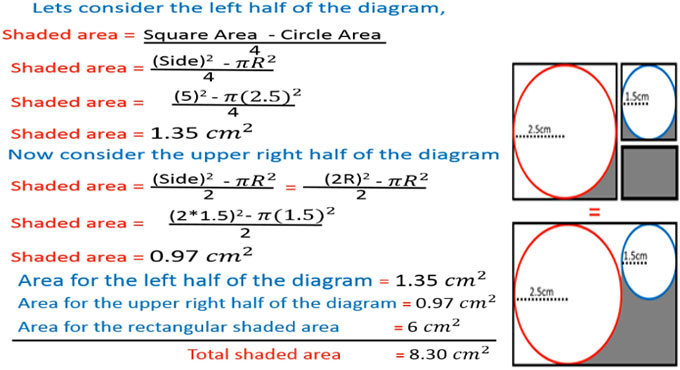
There are two circles in a rectangle. One is small and the other is big. The radius of the big circle is 2.5 cm and the small circle 1.5 cm.
Now, it is required to determine the shaded area of the given diagram.
In the following civil engineering video tutorial, the solution of the above problem is given :-
The shaded area of the left half of the diagram will be calculated with the following formula :-
Shaded Area = Square ? The area of the circle / 4
Shaded Area = (Side)2 ? pR2 / 4 (as one quarter is selected here)
Side of the area = 2R = 2 x 2.5 = 5
After putting the above value, we get the following :-
(5)2 ? p(2.5)2 / 4
Shaded Area = 1.35 cm2
Now, the make the calculation for the upper right half of the diagram as follow :-
Shaded Area = (Side)2 ? pR2 / 2 (as there are two shaded corners)
Shaded Area = (2R)2 - pR2 / 2
Shaded Area = (2 x 1.5)2 - p(1.5)2 / 2
Shaded Area = 0.97 cm2
So, the area of the left of the diagram = 1.35 cm2
and the area of the upper right half of the diagram = 0.97 cm2
For Rectangular Section
The length of the rectangle = Diameter of the small circle = 3 cm
The height of the rectangle = Diameter of the big circle ? diameter of the small circle = 5 ? 3 = 2 = 2 cm
So, the area of the shaded rectangle = 3 cm x 2 cm = 6 cm2
So, the total shaded area = 1.35 + 0.97 + 6 = 8.30 cm2
To get more details, go through the following video tutorial.
Video Source: Engineer Boy